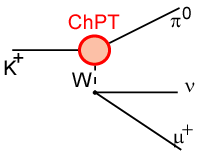
The theory of hadron interaction is quantum chromodynamics (QCD). However, at low energies perturbative methods cannot be applied since the coupling constant becomes very large. One method uses a low energy effective model, Chiral Perturbation Theory (CHPT), which is a rigorous methodology for QCD in the low-energy regions. The predictive power of ChPT can be stringently tested by the form factors of the simplest semileptonic decay K_l3[1]. For the calculation of the K_l3 form factors, a parameterization in terms of the form factors f₊ and f₀ which are associated with vector and scalar exchange, respectively, are convenient. The form factor f₀ is related to f₊ and f_- through

The K_μ3 and K_e3 events were identified by measuring μ⁺s and e⁺s masses, as shown in a figure. The Γ(K_μ3)/Γ(K_e3) ratio can be written as,